
Bryson of Heraclea
Bryson of Heraclea (Greek: Βρύσων, gen.: Βρύσωνος; late 5th-century BCE) was an ancient Greek mathematician and sophist who contributed to solving the problem of squaring the circle and calculating pi.
The name Bryson occurs several times in Aristotle’s writings. A “sophist” Bryson is mentioned as the son of Herodorus of Heraclea (Historia animalium VI, 5, 563a7; IX, 11, 615a10); is blamed for his “sophistic” (in fact, probably proto-Stoic) assertion that there is no such thing as “indecent” language (Rhetoric III, 2, 1405b9); and is blamed for his “eristic” and “sophistic” method of squaring the circle (Posterior Analytics I, 9, 75b40; De sophisticis elenchis 11, 171b16–172a4). A Bryson is named in Plato’s Epistles XIII, where Polyxenus, teacher of Helicon, is designated as his ἑταρος. Theopompus, in Athen XI, 508d (Fragmenta graecorum historicorum, F. Jacoby, ed., 115F259), accuses Plato of having plagiarized Bryson’s Diatribes. It seems preferable (in agreement with Natorp rather than Zeller) to assume that all the above passages refer to one and the same person, although it must be admitted that other biographical information preserved in Diogenes Laertius and Suda, particularly concerning his relations with Socrates, Euclid of Megara, Cleinomachus, Plato, Pyrrho, and Theodorus the Atheist, contain some chronological contradictions.
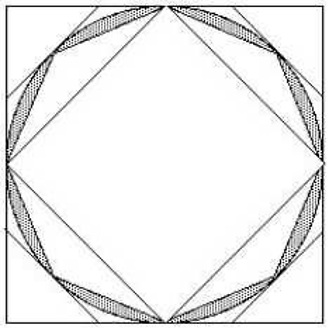
With the help of ancient commentators Bryson’s method of squaring the circle, criticized by Aristotle, can tentatively be reconstructed as follows. We start with two squares, one inscribed in a circle, the other circumscribed. Then we construct successively regular circumscribed and inscribed polygons the perimeters of which approach as closely as we like to the circumference of the circle. Thus, exhausting the area by which one square is larger and the other is smaller than the circle, we eventually make the areas of the larger and the smaller polygon coincide, which means that their areas at that time equal the area of the circle, since the polygon and the circle are both smaller than the circumscribed polygons and greater than all the inscribed figures. Since we can always construct a square equal in area to a polygon, the problem of how to square the circle is solved.
Aristotle criticizes this method because it is based on a principle that is too general and not peculiar to the matter at hand. By this he probably means a principle like this: If two quantities, one larger and one smaller than a third, become equal, they also become equal to that third quantity. It seems that Bryson’s method can, however, be defended against Aristotle and can be considered another anticipation of the principles underlying the method of exhaustion.
No other opinions of Bryson have survived. But since his name is linked with that of Polyxenus, who has been credited with having been the first to put forward the so-called third-man argument, and since Plato was accused of plagiarizing Bryson, it could be that there were similarities between Bryson’s Diatribes and Plato’s Parmenides, in which the third-man argument is indeed discussed.
BIBLIOGRAPHY
In addition to the sources listed in the text, see John Philoponus, In Analytica posteriora, XIII, pt. 3 of Commentaria in Aristotelem Graeca (1909), 111–115, 149; Pseudo-Alexander of Aphrodisias, In Sophisticos elenchos, III, pt. 3 of Commentaria… (1898), 76, 90, 92; and Themistius, Analytica posteriora paraphrasis, V, pt. 1 of Commentaria… (1900), 19–20.
Literature that deals with Bryson includes T. L. Heath, A History of Greek Mathematics, I (Oxford, 1921), 223–225; Paul Natorp, “Bryson 2,” in Pauly-Wissowa, Real-Encyclopädie, III, pt. 1 (1897); W. D. Ross, Aristotle’s Prior and Posterior Analytics (Oxford, 1949), 536 f.; and E. Zeller, Die Philosophie der Griechen, 5th ed., II, pt. 1 (1922), esp. 250, n. 4.
A different interpretation of Bryson’s attempt to square the circle, according to which he proposed establishing some kind of proportion between the circumscribed and the inscribed circles as extremes, the middle term being the square in question, is in A. Wasserstein, “Some Early Greek Attempts to Square the Circle,” in Phronesis, 4 (1959), 92–100, esp. 95–100.
[1]
Sources
[1] "Encyclopedia.com" by Philip Merlan
Our Mobile Application
Check out Our Mobile Application "Ancient Greece Reloaded"


